Conversion of light measurements
Transferred to the new wiki --GW (talk) 15:53, 26 April 2016 (CEST)
Background Information
Simple Lux to magnitudes per arcsec
m_V / [mag per arcsec] = -14.0 + 2.5 log_10(illumination/[Lux]) cf Crawfort 1997
Conversion magnitudes per square arc second to naked eye visual limiting magnitude
The Sky Quality Meter (SQM) measures sky brightness and expresses it as the logarithm of a ratio to the brightness of standard stars - the usual astronomical magnitude system. For a conversion to naked eye visual limiting magnitude and other quantities, see the recommendations of the manufacturer (Unihedron):
They quote:
Naked eye limiting magnitude in zenith [mag] =7.93-5*log(10^(4.316-((SQM-reading/[mag/"²])/5))+1)
and for the inverse
NELM=7.93-5*log(10^(4.316-(Bmpsas/5))+1)
This formula should be applied with care and taking into accout that SQM-readings include a large fraction of sky that may contain extra sources as the milky way or bright stars that can easily cause 0.3 mag differences in SQM-measurements that are not relevant for lim. magnitude.
Various Conversions
Limiting Magnitude Calculations hacked together by Ben Sugerman
Beleuchtungsstärke, Himmelshelligkeit, Grenzgröße
Ich hab' einmal versucht, eine ungefähre Umrechnung der Beleuchtungsstärke (Lux) in die Himmelshelligkeit (mag pro Quadratbogensekunde) und die freiäugige Grenzgröße zu finden. Unter der Annahme, ein dunkler Nachthimmel hat eine Beleuchtungsstärke von 0,002 Lux [1] und eine Himmelshelligkeit von 22mag/Quadratbogensekunde lässt sich die Formel für die Umrechnung der Beleuchtungsstärke in die Himmelshelligkeit folgendermassen herleiten:
0,002 Lux = 22mag/Quadratbogensekunde
1 Lux = 500 * 22mag/Quadratbogensekunde
1 Lux = -6,78 + 22 mag/Quadratbogensekunde
1 Lux = 15,22mag/Quadratbogensekunde
Die Formel für die Umrechnung lautet somit:
Himmelshelligkeit [mag/Quadratbogensekunde] = 15,2 - ln(Lux)/ln(2,5)
Trifft man als weitere Annahme, dass der Himmel mit 22mag/Quadratbogensekunde einer freiäugige Grenzgröße von 6,0mag entspricht, gilt:
Grenzgröße [mag]= Himmelshelligkeit - 16,0
Wie erwähnt gelten die obigen Formeln für die von mir getroffene Annahme, dass eine Beleuchtungsstärke von 0,002 Lux einer Himmelshelligkeit von 22mag/Quadratbogensekunde und einer feiäugige Grenzgröße von 6,0mag entsprechen. Sollten sich aus der Praxis andere Werte ergeben, kann man das durch Änderung der Konstanten einfach anpassen. --HerbertRaab 23:46, 29. Aug. 2008 (UTC)
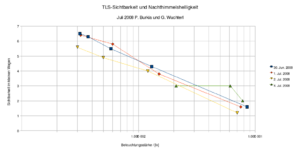
Visual limiting magnitude as function of horizontal illuminance
Fit to the sightings of P. Burkia according to the "How many stars...?"-images (HMS) and parallel measurements with the Libelle 2 Tautenburg Lightmeter on June 30th 2008.
vis mag = -1.49 ln(Lux) -2.11 -0.5; R² = 1.0
The extra term of -0.5 mag is a rough way to compensate for the centering of HMS-UMi images that have to rely on exisiting stars with respect to the visual limiting magnitudes. E.g. the brightest indicator-star for image 7 of HMS is about 6.6 mag). Use of the formula at limiting magnitudes above 7 should be carefully considered as the HMS-method ends at 7mag.
--GuentherWuchterl 15:44, 30. Okt. 2010 (UTC)
Scheinbare Helligkeiten [mag] und Beleuchtungsstärke [lx]
Umwandlung von Magnituden [mag] nach Lux [lx]
Apparent brightness of single sources on the sky and the illuminance caused when they are shining from zenith.
From Lux-measurments and tabulated magnitudes of the Sun and the typical (full) Moon Aus Luxmessungen und Magnituden von Sonne oder Mond [2]
Illuminance (Beleuchtungsstärke) [lx] = 10.0**(-0.40195*mag-5.5917)
Fit von André Müller:
Illuminance (Beleuchtungsstärke) [lx] = 2.54E-6 * 10^( -0.4 * mag )
Comparison/Vergleich:
-12.5mag Müller 7% grösser
6mag Müller 2% kleiner
Zu mag/Quadratbogensekunden nach Lux
Die Magnituden pro Quadratbogensekunde hängen mit der Leuchdichte (des Himmels) zusammen. Sie sind ein Logarithmus eines Verhältnisses der Himmelsleuchtdichte zu einer Standardleuchtdichte.
Leuchtdichte
In SI Einheiten wird die Leuchtdichte is candela pro Quadratmeter, [cd/m²] angegeben
Conversion mag/arcsec² to cd/m²
Recommended by for the SQM by Unihedron [3]:
[value in cd/m2] = 10.8×10^4 × 10(-0.4*[value in mag/arcsec2])
Typische Leuchtdichten [cd/m2] (nach einem Vortrag von Thomas Posch)
Integriertes Licht aller Sterne bis 21mag 83 ucd/m2 mikro candela pro Quadratmeter dunkelster je registrierter Himmel 171 Natürlicher Himmelshintergrund NHH 250 IAU Comm 50 Himmelverschmutzt wenn > 275 bei h>45 Grad (1.1 NHH) Sichtbarkeit der Milchstrasse < 3700
Von Leuchtdichte zu Beleuchtungsstärke:
Integral von Leuchtdichten L über die Halbkugel zur Berechnung von Lux
Int L sin h cos h d A d h (A Azimut, h Höhe) ; sin h Projektion auf
mit Leuchtdichte L beleuchtete horizontale
Ebene.
über h = [0,pi/2], A = [0.2pi ] ()Hemisphäre = 1/2 cos² h|_0^pi/2 = pi!
Isentroper Emitter mit Leuchtdichte L aus Hemisphäre (zB Nachthimmel mit konstanter Leuchtdichte):
Beleuchtungsstärke [lx] = pi L [cd/m²]
Von Quadratbogensekunden zum gesamten Himmel
Umrechnungsfaktor von Quadratbogensekunde auf die Himmelshalbkugel:
Bogensekunde vs Bogenmass: 360 * 60 * 60 ["] = 2 pi [rad]
--> [rad] = 360 * 60 * 60 / ( 2 pi ) ["]
[mag/"2] = [mag/ 360 * 60 * 60 / ( 2 pi )²]
[mag/rad²] = ( 360 * 60 * 60 / ( 2 pi ) ) * [mag/"2]
= 4.25452E10 [mag/"2]
[/hemisphäre] = 90 * 360 * 60² * 60² [/"²]
Umrechnungsfaktor umgerechnet in Magnitudendifferenz:
[/hemisphäre] / [/"²] = -29.1 mag Flächenverhältnis der Halbkugel zur Quadratbogensekunde
Ergibt mit der Umrechnung von mag in lx zwei Faustregeln für ...
Berechnung der Beleuchtungsstärke eines Himmels mit bestimmten mag/²"
Calculation of the horizontal illuminance [measured in Lux] caused by a sky (one hemisphere) with a constant luminance [according to SI measured in cd/m²] but expressend here in magnitudes per square arcsecond, we have as a proxy for the decadic logarithm of the horizontal illuminance: --GW (talk) 16:22, 26 April 2016 (CEST)
lg Lux = 6 - 2/5 * mag/²" Dabei ist lg ist der dekadische Logarithmus.
and the inverse relation between Luminance and the horizontal illuminance on the ground beyond a hemisphere (sky of constant luminance:
Luminance [mag/²"] = - 5/2 lg Illuminance [Lux] + 15
Genau genommen ist es die horizontale Beleuchtungsstärke auf Boden oder Luxmeter die ein Himmel (Halbkugel) mit konstanter Leuchtdichte die mag/²" entspricht ergibt.
Irradiance and Energy Flux-Density: Lux and W/m^2
Aus Solarkonstante 1368 W/m^2 und 130 000 lx für Sonne und Zenit bastle ich
[Lux] = 95.02 [Watt/m^2]
das bedeutet: 1 Lux = 0.001523 W/m^2
Habe jetzt auch gerade gesehen dass photopische und scotopische lumen zu entsprechenden Luxen führen. Das monochromatische Watt is 680 bzw. 1720 Lumen, das allein reicht mir zum Abschied vom Lux der mit Deiner Kalibration ja jetzt leicht fällt.
